|
|
|
|
| ||
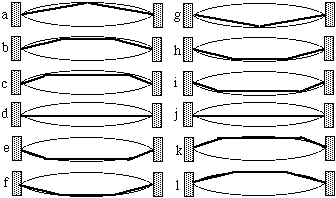
A sketch of the reflection of travelling kinks caused by plucking a string. The bold line represents the string, and the thin line is the path traced out by the kink. At the instants represented by (d) and (j), the string is straight so it has lost the potential energy associated with pulling it sideways, but it has a maximum kinetic energy. Note that, at the reflections, the phase of the kink is changed by 180°: from up to down or vice versa. Notice also how the kinks 'pass through' each other when they meet in the middle.
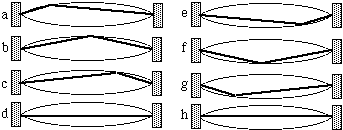
A sketch of the reflection of travelling kinks caused by bowing a string. See the animation of the bow-string interaction in Bows and strings
Why is the reflection inverted? Well, if we assume that it is clamped or tied to a fixed object, the point of reflection didn't actually move. But look at the motion of the string by comparing the different times represented in the left hand sketches. Note that the string behind the kink is moving back towards the undisturbed position (down in the sketch). As the kink approaches the end, it becomes smaller and, when it reaches the immovable end, there is no kink at all - the string is straight for an instant. But the string still has its downwards momentum, and that carries it past the position of rest, and produces a kink on the other side, which then moves back in the other direction .
|
An interesting effect occurs if you try to send a simple wave along the string by repeatedly waving one end up and down. If you have found a suitable rubber hose, try it out. Otherwise, look at the diagram at right. The figure represents a time sequence - time increases from top to bottom. You could think of it as representing a series of photographs of the waves, taken very quickly. The blue wave is travelling to the right, the green to the left. The red line is their sum: the red wave is what happens when the two travelling waves add together (superpose is the technical term), so the red wave is what we would actually see in a photograph - the blue and green are the two waves that are interacting. Suppose that the right hand limit is an immoveable wall. As discussed above the wave is inverted on reflection so, in each "photograph", the blue plus green adds up to zero on the right hand boundary. The reflected (green) wave has the same frequency and amplitude but is travelling in the opposite direction. At the fixed end they add to give no motion - zero displacement: after all it is this condition of immobility which causes the inverted reflection. But if you look at the red line on the diagram (representing the sum of the two waves) you'll see that there are other points where the string never moves! They occur half a wavelength apart. These motionless points are called nodes of the vibration, and they play an important role in all of the instrument families. Halfway between the nodes are antinodes: points of maximum motion. But note that these peaks are not travelling along the string: the combination of two waves travelling in opposite directions produces a standing wave. This is shown in the figure. Note the positions (nodes) where the two travelling waves always cancel out, and the others (antinodes) where they add to give an oscillation with maximum amplitude. You could think of this diagram as a representation of the fifth harmonic on a string whose length is the width of the diagram. This brings us to the next topic. |
|
The string on a musical instrument is (almost) fixed at both ends, so any vibration of the string must have nodes at each end. Now that limits the possible vibrations. For instance the string with length L could have a standing wave with wavelength twice as long as the string (wavelength = 2L) as shown in the first sketch in the next series. This gives a node at either end and an antinode in the middle.
This is one of the modes of vibration of the string ("mode of vibration" just means style or way of vibrating). What other modes are allowed on a string fixed at both ends? Several standing waves are shown in the next sketch.
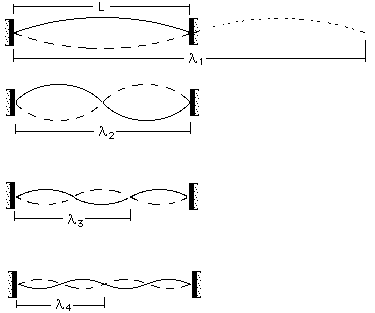
A sketch of the first four modes of vibration of an idealised* stretched string with a fixed length. The vertical axis has been exaggerated.
Let's work out the relationships among the frequencies of these modes. For a wave, the frequency is the ratio of the speed of the wave to the length of the wave: f = v/wavelength. Compared to the string length L, you can see that these waves have lengths 2L, L, 2L/3, L/2. We could write this as 2L/n, where n is the number of the harmonic.
The fundamental or first mode has frequency f1 = v/wavelength =
v/2L,
The second harmonic has frequency f2 = v/l2 = 2v/2L = 2f1
The third harmonic
has frequency f3 = v/l3 = 3v/2L =
3f1,
The fourth harmonic has frequency f4 = v/l4 = 4v/2L = 4f1, and, to generalise,
The nth harmonic has frequency fn = v/ln = nv/2L = nf1.
All waves in a string travel with the same speed, so these waves with different wavelengths have different frequencies as shown. The mode with the lowest frequency (f1) is called the fundamental. Note that the nth mode has frequency n times that of the fundamental. All of the modes (and the sounds they produce) are called the harmonics of the string. The frequencies f, 2f, 3f, 4f etc are called the harmonic series. This series will be familiar to most musicians, particularly to buglers and players of natural horns. If for example the fundamental is the note C3 or viola C (a frequency of 131 Hz), then the harmonics would have the pitches shown in the next figure. These pitches have been approximated to the nearest quarter tone. The octaves are exactly octaves, but all other intervals are slightly different from the intervals in the equal tempered scale.
| The figure shows the musical notation for the first twelve
harmonics on a C string. When you play the sound file, listen carefully to
the pitch. The seventh and eleventh harmonics fall almost halfway between
notes on the equal tempered scale, and so have been notated with half
sharps.
|
|
(* If you have just done this experiment, you may have noticed some peculiarities. The twelfth fret, which is used to produce the octave, is less than half way along the length of the string, and so the position where you touch the string to produce the 2nd harmonic is not directly above the octave fret. I said "idealised" string above, meaning a string that is completely flexible and so can bend easily at either end. In practice, strings have a finite bending stiffness and so their effective length (the "L" that should be used in the above formulae) is a little less than their physical length. This is one of the reasons why larger strings usually have a winding over a thin core, and why the G string on a classical guitar has poor tuning on the higher frets. There is also an effect due to the extra stretching of a string when it is pushed down to the fingerboard, an effect which is considerable on steel strings.)
An exercise for guitarists. On a guitar tuned in the usual way, the B string and high E string are approximately tuned to the 3rd and 4th harmonics of the low E string. If you pluck the low E string anywhere except one third of the way along, the B string should start to vibrate, driven by the vibrations in the bridge from the harmonic of the first string. If you pluck the low E string anywhere except one quarter of the way along, the top E string should be driven similarly.
| Guitarists often begin to tune-up in the following way:
first tune the 4th harmonic of the low E string, the 3rd of the A string
and the top E all to the same note. The figure at right shows the harmonic
series on the two lowest strings.
Next they tune the B string (B3) to the 3rd harmonic of the first (E2); then tune the 4th harmonic of the A string to the 3rd of the D string. This method cannot be extended succesfully to the G string because it is usually too thick and stiff, so it is better tuned by octaves, using the frets. For several reasons (see the notes at the end of this page), this method of tuning is only approximate, and one needs to retune the octaves afterwards. The best tuning is usually a compromise that must be made after considering what chords you will be playing and where you are playing on the fingerboard. |
|
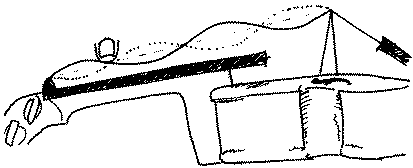
Harmonics in music. Composers often call for such harmonics on string instruments: the most common is the "touch fourth";. With one finger, the player stops the string to produce the length required for a particular note, and then, using another finger, touches the string very lightly at the position required for the note four notes higher in the scale (hence the name). This position is one quarter of the way along the string, so it produces the fourth harmonic of the stopped note. The fourth harmonic has four times the fundamental frequency, and so is two octaves higher. For string players, the harmonics are called "natural"; when they are played on open strings and "artificial"; if the player must stop the string. The diagram shows the how a natural touch fourth is played, and the notation for the touch fourth on the violin A string. The vertical axis of the diagram has been exaggerated for clarity.

![]()
![]() Open A string played normally, then the touch fourth on this
string (4th harmonic)
Open A string played normally, then the touch fourth on this
string (4th harmonic)
The pitch of a note is determined by how rapidly the string vibrates. This depends on four things:
We can put all of this in a simple expression. If the vibrating part of the string has a length L and a mass M, and if the tension in the string is F and if you play the nth harmonic, then the resulting frequency may be written as (n/2L)(FL/M)1/2 or (n/2)(F/LM)1/2. In instruments such as the violin and guitar, the open length and the tension are fairly similar for all strings. This means that, to make a string an octave lower, you must quadruple the ratio M/L. If the strings are made of the same material, this means doubling the diameter. However, the fat strings are usually composite: a thin core wrapped with windings to make them more massive without making them harder to bend.
For more information, see
| Some technical information for string players. How
do you work out harmonics if they are not explicitly annotated? Although
the touch fourth is the most common harmonic, it has a disadvantage as an
example. A touch fourth produces the fourth harmonic, but the two
"fourth"s are from quite different context. In no other simple case does a
touch nth produce the nth harmonic. For the low harmonics, the rule is
obvious: 1/n of the string produces the nth harmonic. This formula starts
to fail at very high numbers where the finite thickness of the string is
important. Further, it is not a reliable way of producing harmonics above
about the 8th.
String players will know that, if you play five scale notes up a string, you arrive at a position one third of the way along the string, so a "touch fifth" produces the third harmonic. We can write the harmonics in the format: Scale . . . . . fraction . . harmonic . interval octave . . . . . 1/2 . . . . . 2. . . . . . octave |
|
The scale positions are in just intonation. The touch at 2/9 is safer than that at 1/9, but it doesn't fall above any scale note position: it is a little above the minor third. Violists or violoncellists rehearsing Radulescu's "Practicing Infinity" (sic) are invited to write to me for further suggestions about techniques for high harmonics.
See also How harmonic are harmonics?
Complications with harmonic tuning. There are several problems with any guitar tuning, including that using harmonics suggested above.
The most obvious approximation is related to temperament: if the guitar strings were ideal and the frets ideally spaced for equal temperament, tuning harmonic fourths to the E-A and A-D pairs, plus two equal tempered semitones on the D string, would make the interval between lowest E and 2nd fret on the D string about 4 cents flat ((4/3)222/12=1.996). This would lead to interference beats at rates of order one every several seconds.
Another obvious complication with harmonic tuning is that the strings do not bend with complete ease over the nut and bridge (as discussed above). See also How harmonic are harmonics.) As a result, the 1st overtone on a string is slightly sharper than an octave, the next even sharper than a twelfth, and so on. So tuning the 4th 'harmonic' of the E string to the 3rd of the A string makes them their open interval more than a harmonic fourth. So this tends to compensate for the temperament problem.
A further problem has to do with fret and bridge placement. When you press a string down at the twelfth fret, you increase its length. (Before you press it, the shortest distance between nut and bridge. Afterwards it is longer.) To lengthen it, you have increased its tension. Because of this, and also because of the bending effect at the end of the string, if the 12th fret were midway between nut and bridge, the interval would be greater than an octave. (You can check this experimentally on a fretless instrument.) Consequently, the distance from bridge to the 12th fret is greater than that from the nut to the 12th fret. The effect differs among strings. In some electric guitars, individual adjustment of the position of each bridge is possible. In other guitars, the bridge is placed at an angle. In a classical guitar, the straight simple bridge necessitates some compromise in tuning.
The effects above are difficult measure with experimentally with the required precision: the effects are only a few cents, which is not much larger than the precision of ears or tuning meters when applied to a pluck string. Further, it is difficult to adjust machine heads to achieve a precision better than a couple of cents. On the other hand, if you get all notes in tune within a couple of cents, you are doing better than most musicians and it will sound pretty good!
There are further problems when strings get old. Where you finger them with the left hand, they pick up grease and become more massive (although they may also lose material where they rub on frets). They may also wear where you pick them. As the strings become inhomogeneous, the tuning gets successively worse. Washing them can help.
The way to get around most of these problems is to play fretless instruments, but this makes chords more awkward.
|
|
| * This figure shows the harmonics on the open G string of
either the cello or the bass. (Remember that bass music is written one
octave higher than it sounds.) Above the notes are written the harmonic
numbers, and below are written the approximate frequencies in Hertz
(they have been rounded off to make the arithmetic more obvious.)
This diagram shows the logarithmic nature of your sense of pitch: although the frequency increases by the same absolute change of 100 Hz, the proportional change becomes successively smaller, as does the pitch. |
|
|